Question 1 (a)

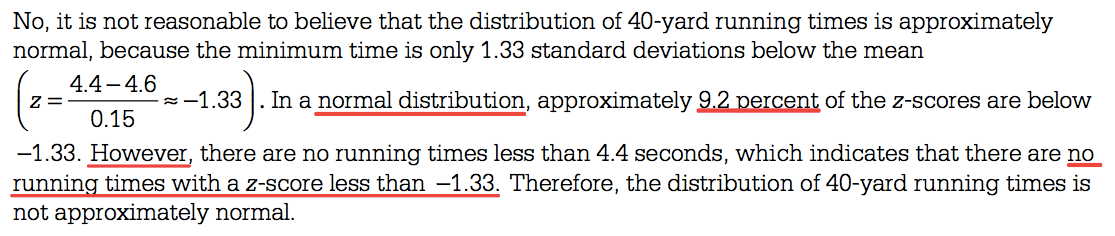
No, it is not reasonable to believe that the distribution of 40-yard running times is approximately normal, because the minimum time is only 1.33 standard deviations below the mean 4.4—4.6 —1.33 . In a normal distribution, approximately of the z-scores are below 0.15 —1.33. However there are no running times less than 4.4 seconds, which indicates that there are no running times with a z-score less than —1.33. Therefore, the distribution of 40-yard running times is not approximately normal.

Question 1 (b)

  • How to interpret the z-score

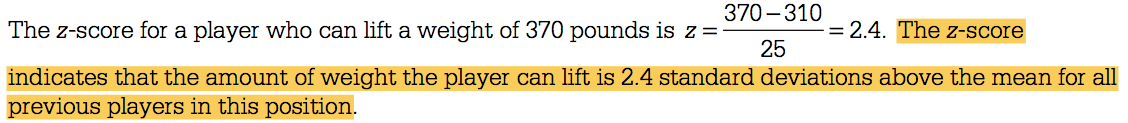
    370-310 The z-score for a player who can lift a weight of 370 pounds is z = 25 Indicates that the amount o weight the player canlift IS 2.4 standard deviations above the meanforall previous players in this position.

Question 2 (b)

  • Independence vs. Dependence

    • A conditional probability is the probability of some event occurring, given that some other event has already occurred. The conditional probability of event X occurring, given that some other event Y has already occurred, is written as P(X|Y).

    • For example, P(M|N) would be the probability of the occurrence of event M given that event N has already occurred. It would be read as “the probability of M, given N.”

    • As stated earlier, two events are considered independent if the occurrence of one of the events does not change the probability of the other event from what it would have been had the first event not occurred. Thus, two events, X and Y, are independent if P(X|Y) = P(X) or P(Y|X) = P(Y)

    • Actually, these two conditional relationships are related. If one is true, the other must be true. If one is false, the other must be false.

    • If P(X|Y) = P(X), then P(Y|X) = P(Y), and the events are independent.

    • If P(X|Y) ≠ P(X), then P(Y|X) ≠ P(Y), and the events are dependent.

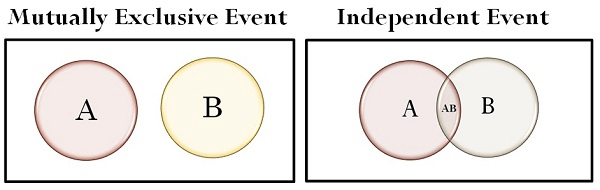
    Mutually Exclusive Event Independent Event

Question 2 (c)

The marginal proportions of voters registered for each of the three political parties (without regard to gender) are given below. 88 =0.176 Party W: 500 244 = 0.488 Party X: 500 168 = 0.336 Party Y: 500 Because party registration is indeoendent of aender in Lawrence Township, the proportions of males and females registered for each party must be identical to each other and also identical to the marginal proportion of voters registered for that party. Using the order Party W, Party X, and Party Y, the graph for Lawrence Township is displayed below.

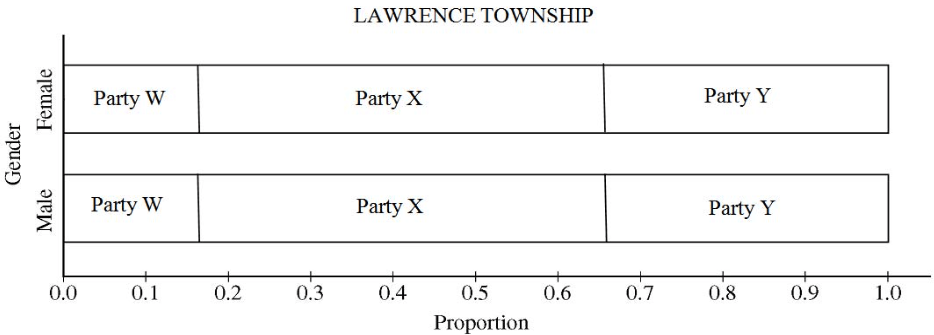
0.0 Party W Рапу W 0.1 0.2 0.3 I.AWRENCE TOWNSHIP Party Х Party Х 0.4 0.5 0.6 Proportjon 0.7 Рапу У Party У 0.8 0.9 1.0

Question 3 (a)

  • Process for randomly selecting 2 numbers from 1 to 9

    Step 1: Generate a random integer between 1 and 9, inclusive, using a calculator, a computer program, or a table of random digits. Select all four apartments on the floor corresponding to the selected integer. Step 2: Generate another random integer between 1 and 9, inclusive. If the generated integer is the same as the integer generated in step 1 , continue generatinq random inteqers between 1 and 9 until a different integer appears. Again select all four apartments on the floor corresponding to the second selected integer.

Question 3 (b)

Because the amount of wear on the carpets in apartments with-GhiIdK.en-GQ.uld-he-diffeI.ent.ft.Q111 the wear on the carpets in apartments without children, it would be advantageous to have apartments with children represented in the sample. The cluster sampling procedure in part (a) could produce a sample with no children in the selected apartments; for example, a cluster sample of the apartments on the third and sixth floors would consist entirely of apartments with no children. Stratified random sampling, where the two strata are apartments with children and apartments without children, guarantees a sample that includes apartments with and without children, which, in turn, would yield sample data that are representative of both tvpes of apartments.

Question 4

  • Degrees of freedom for two-sample t-test.

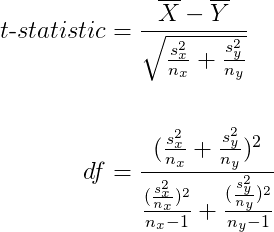
    %-statistic — (4)2

  • Graphs of both distributions must be produced and described to check the normality condition.

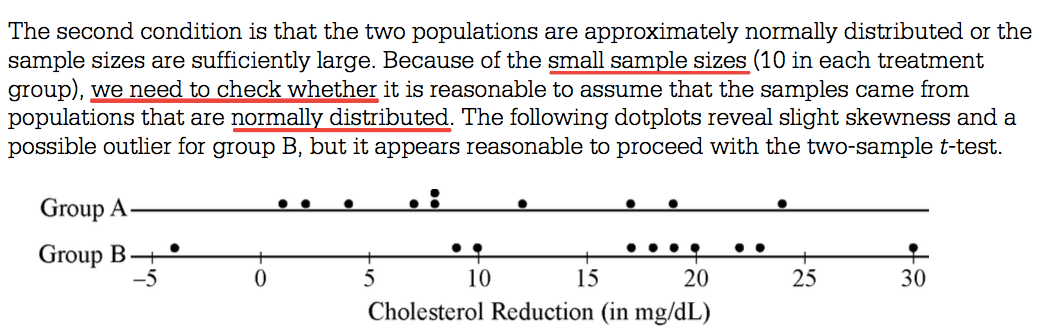
    The second condition is that the two populations are approximately normally distributed or the sample sizes are sufficiently large. Because of the small sample sizes (10 in each treatment group), we need to check whether it is reasonable to assume that the samples came from populations that are normally distributed. The following dotplots reveal slight skewness and a possible outlier for group B, but it appears reasonable to proceed with the two-sample t-test. Group A Group B -5 5 10 15 20 25 30 Cholesterol Reduction (in mg/dL)

Question 5 (c)

  • Correlation coefficient

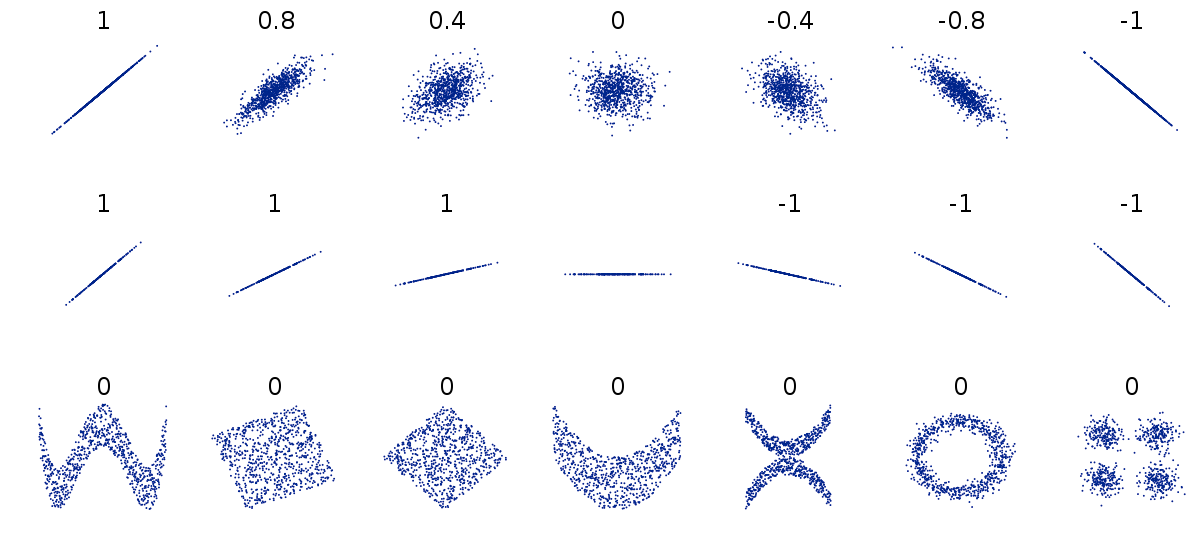
    C:\\6432CA65\\FE01530B-89BD-4F8B-A3E1-55F12080AD12\_files\\image338.png

  • Coefficient of determination

    The coefficient of determination (denoted by R2) is a key output of regression analysis. It is interpreted as the proportion of the variance in the dependent variable that is predictable from the independent variable. An R2 between O and 1 indicates the extent to which the dependent variable is predictable. Coefficient of Determination: Definition - Stat Trek Prot onlinecourses.science.psu.edu stattrek.com/statistics/dictionary.aspx?definition=coefficient\_of\_determination

Question 5 (d)

  • Explaining relationship

    Yes, there is very strong statistical evidence that the population slope differs from zero, so electricity production is linearly related to wind speed. For testing the hypotheses Ho : ß = 0 versus Ha : 0, where represents the population slope, the output reveals that the test statistic is t = 12.63 and the p-value (to three decimal places) is 0.000. Because (much less than both 0.05 and 0.01), the sample data provide that electricity production is linearly related to wind speed.

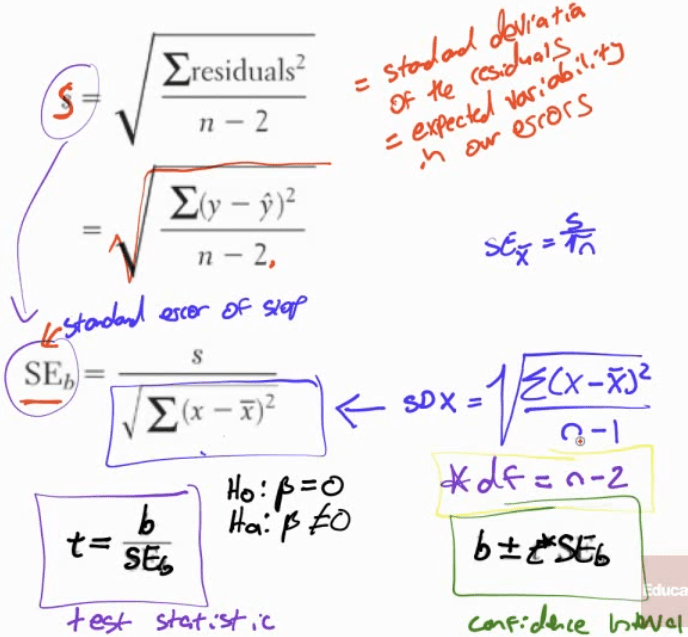
    n 2— n ,2— z»oegq-o «را ود - ن •ى (T) مى ى ؛ملا ه ت على ت 2-٥ لاتوج هحاe،ع:مى

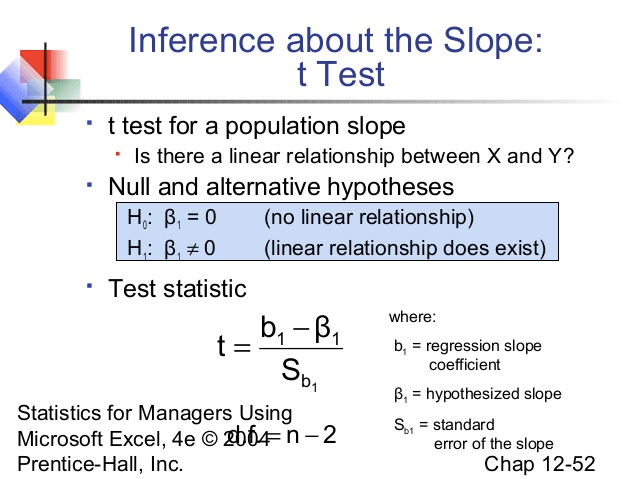
    Inference about the Slope: t Test t test for a population slope Is there a linear relationship between X and Y? Null and alternative hypotheses 131 = o (no linear relationship) linear relationshi does exist Test statistic where: Statistics for Managers Using Microsoft Excel, 4e O n -2 Prentice-Hall, Inc. b, = regression slope coefficient = hypothesized slope So 1 = standard error of the slope Chap 12-52

Question 6 (a)

  • Checking condition for one-sample z-interval

    • Random

    • Normal: Large sample size

    To satisfy the third component, the response: o Must check o Must use a reasonable criterion (for example, 5 or 210). o Must provide numerical evidence (for example, 2,688 210 and 10, or 9,600x0.28210 and 10) .

results matching ""

    No results matching ""